Samples and surveys worksheets with answers
Table of Contents
Table of Contents
In the world of statistics and data analysis, the line of best fit is an essential concept. It enables us to interpret data easily and predict outcomes with accuracy. But, how to draw best fit line?
When working with data, we often have scattered points, and it can be challenging to determine a pattern. However, as humans, we are wired to find relationships and patterns. But, in most cases, we need to have the line of best fit, which summarizes the trend in data, to make meaningful conclusions.
The line of best fit refers to a straight line that best represents the trend in the data. It minimizes the distance between the points and the line. A line of best fit can help provide insight into the relationship between variables and make predictions with minimal errors.
To draw the line of best fit, we use a slightly more complicated method called linear regression. This method involves finding the slope and y-intercept of the line that minimizes the distance between the line and the data points.
My Personal Experience with Drawing the Line of Best Fit
As a data analyst, drawing the line of best fit is an essential skill that I have honed over time. One experience that stands out was when I had to analyze sales data for products from our company’s website. The data was scattered, and without the line of best fit, it was nearly impossible to determine the overall trend.
I followed the steps to find the line of best fit, and to my surprise, the line fit almost perfectly with the rest of the data points. From this line, I could deduce that the sales of the product were increasing, and I could predict future sales accurately.
How to Draw the Line of Best Fit
The first step in drawing the line of best fit is to plot all the data points on a scatter plot. Then, we need to determine the slope and y-intercept of the line. Typically, there are two methods for calculating the line of best fit; the Least Square Method and Gradient Descent Method.
The Least Square Method
The Least Square Method is the most common method for finding the line of best fit. In this method, we try to minimize the sum of the squares of the distances between the points and the line.
First, we find the mean of both the x and y values. Then, for each point, we calculate the difference between the x-coordinate and the mean of the x-coordinates, as well as the difference between the y-coordinate and the mean of the y-coordinates. We then multiply these two differences for each point and find the sum of all these products.
Next, we need to calculate the sum of the squares of the differences between the x-coordinate and the mean of the x-coordinates.
Finally, we calculate the slope of the line by dividing the sum of the products by the sum of the squares of the differences between the x-coordinates and their mean. Similarly, we can calculate the y-intercept by using the following formula:
Y-Intercept = Mean of y-coordinates - (slope * Mean of x-coordinates)
The Gradient Descent Method
The Gradient Descent Method involves finding the slope and y-intercept of the line iteratively. In this method, we randomly initialize the slope and y-intercept of the line, and then we keep updating these values until we reach the minimum difference between the line and the data points.
Conclusion of How to Draw Best Fit Line
The line of best fit is essential in data analysis, and it helps us make predictions and get insight into the relationship between variables. Drawing the line of best fit is relatively easy as long as you know the steps. There are different methods to finding the line of best fit, including the Least Square Method and Gradient Descent Method.
Question and Answer
Q. Is the line of best fit always the same for all data points?
A. No, the line of best fit is unique for different datasets. Every dataset has a distinctly different pattern, and the line of best fit follows the pattern in the dataset.
Q. Can the line of best fit be a curve?
A. No, the line of best fit is always a straight line. However, in some cases, it may appear curved due to scaling issues in the axes.
Q. Why is the line of best fit important?
A. The line of best fit summarizes the trend in the data and enables us to make meaningful conclusions. It helps provide insight into the relationship between variables and makes predictions with minimal errors.
Q. Can the line of best fit be used for non-linear relationships?
A. No, the line of best fit can only be used for linear relationships. For non-linear relationships, we need to use other methods such as quadratic regression or exponential regression.
Gallery
Constructing A Best Fit Line
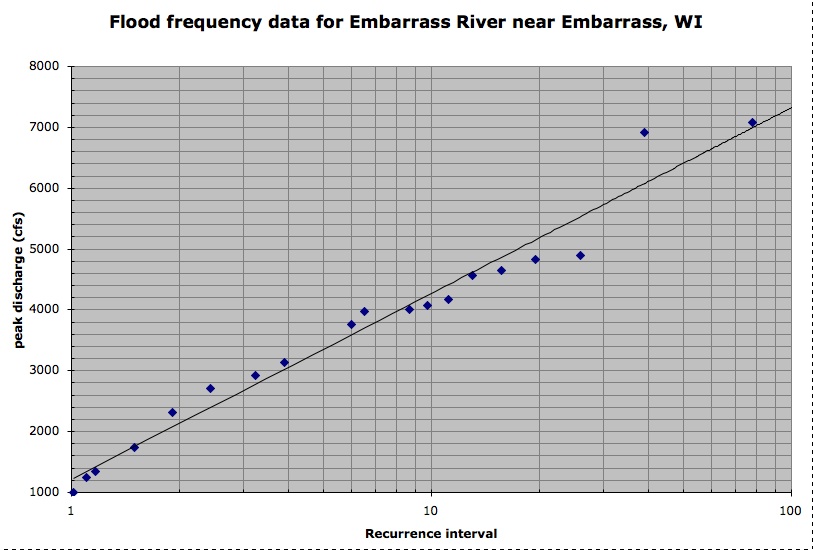
Photo Credit by: bing.com / line fit lines should when bestfit most use construction methods many
How To Draw A Line Of Best Fit - YouTube

Photo Credit by: bing.com / line fit draw scatter lines plots
Samples And Surveys Worksheets (With Answers) | Cazoom Maths

Photo Credit by: bing.com / scatter graphs worksheet maths lines worksheets plots trend line fit math answers graph statistics science plot surveys resources teaching example
How To Find The Line Of Best Fit In 3 Steps

Photo Credit by: bing.com / tutorme tutoring
How To Draw A Line Of Best Fit - YouTube
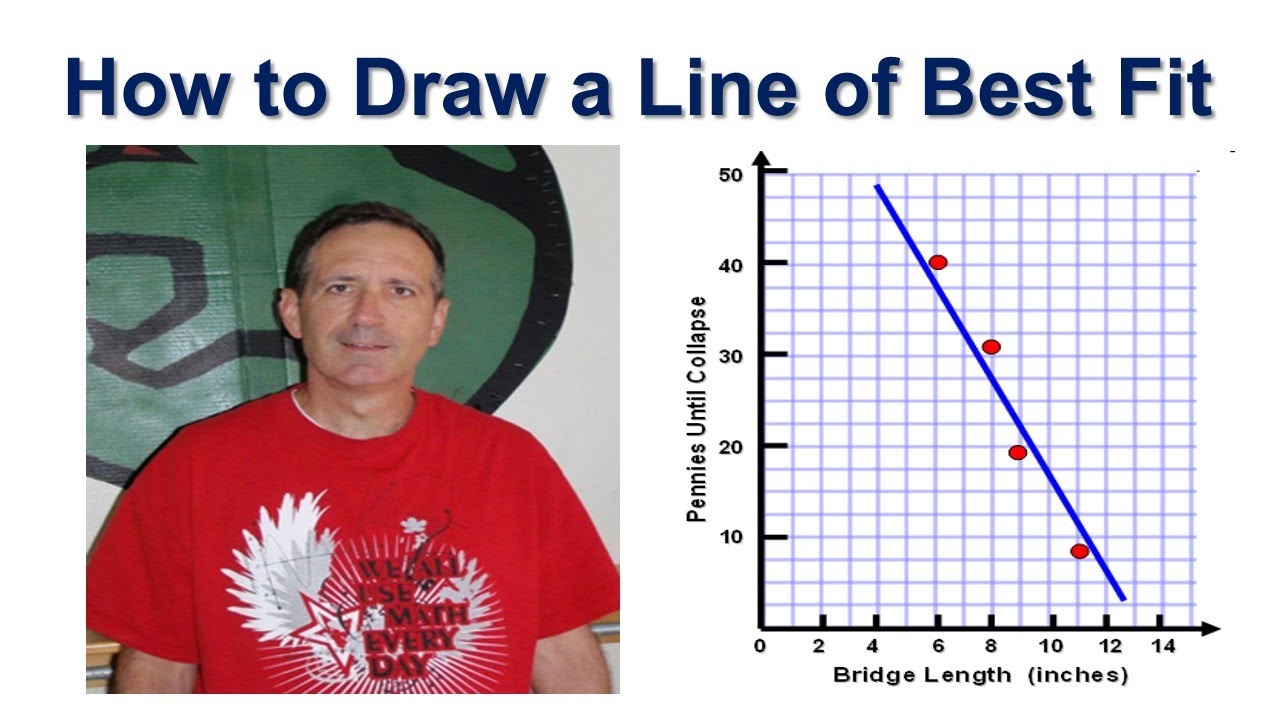
Photo Credit by: bing.com / line draw fit






